Moench Solution for Double-Porosity Fractured Aquifers

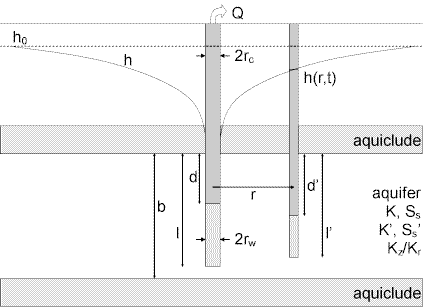
Moench (1984) derived an analytical solution for unsteady flow to a fully penetrating, finite-diameter well with wellbore storage and wellbore skin in an isotropic fractured aquifer assuming a double-porosity model with slab or spherical matrix blocks and fracture skin

Moench (1988) extended the method to include partially penetrating wells and anisotropy based on the solution by Dougherty and Babu (1984). When you choose the Moench solution in AQTESOLV, you may analyze data for fully or partially penetrating wells. The solution also includes delayed response in an observation well based on the work of Moench (1997).

AQTESOLV provides two configurations for simulating a double-porosity aquifer using the Moench (1984) solution: slab-shaped and spherical matrix blocks. Select the matrix option when you choose a solution.

You are not restricted to constant-rate tests with the Moench solution. AQTESOLV incorporates the principle of superposition in time to simulate variable-rate and recovery tests with this method.

Wellbore storage has a distinct signature in the early-time response of a pumped well. Use the radial flow and derivative-time plots to detect the wellbore storage effect.

Assumptions

• aquifer has infinite areal extent
• aquifer has uniform thickness
• aquifer potentiometric surface is initially horizontal
• wells are fully or partially penetrating
• fractured aquifer represented by double porosity system consisting of low-permeability, primary porosity blocks and high-permeability, secondary porosity fissures
• matrix consists of slab-shaped or spherical blocks
• flow is unsteady
• water is released instantaneously from storage with decline of hydraulic head

Equations

Moench (1984) derived an analytical solution for flow to a finite-diameter pumping well with wellbore skin in a double-porosity fractured aquifer assuming slab-shaped or spherical matrix blocks and fracture skin.

The Laplace transform solution for dimensionless drawdown in the pumped well is as follows:

$${\overline{h}}_{wD}=\frac{2\left[K_0\left(x\right)+x{S}_w{K}_1\left(x\right)\right]}{p\left\{p{W}_D\left[K_0\left(x\right)+x{S}_w{K}_1\left(x\right)\right]+x{K}_1\left(x\right)\right\}}\text{(1)}$$

The following equation is the Laplace transform solution for dimensionless drawdown in an observation well:

$${\overline{h}}_D=\frac{2K_0\left(r_{D}x\right)}{p\left\{p{W}_D\left[K_0\left(x\right)+x{S}_w{K}_1\left(x\right)\right]+x{K}_1\left(x\right)\right\}}\text{(2)}$$ $$x=\sqrt{p+{\overline{q}}_D}\text{(3)}$$ $${\overline{q}}_D=\frac{{\gamma }^{2}m\tanh \left(m\right)}{1+S_{f}m\tanh \left(m\right)}\text{slab blocks}\text{(4)}$$ $${\overline{q}}_D=\frac{{3\gamma }^2\left[m\coth \left(m\right)-1\right]}{\left\{1+S_f\left[m\coth \left(m\right)-1\right]\right\}}\text{spherical blocks}\text{(5)}$$ $$m=\frac{\sqrt{\sigma p}}{\gamma }\text{(6)}$$ $$\gamma =\frac{{2r}_w}{b^{\prime }}\sqrt{\frac{K^{\prime }}{K}}\text{(7)}$$ $$\sigma =\frac{S_s^{\prime }}{S_s}\text{(8)}$$ $$r_D=r/r_w\text{(9)}$$ $$S_f=\frac{{2K}^{\prime }{b}_s}{K_s{b}^{\prime }}\text{(10)}$$ $$W_D=\frac{\pi r_c^2}{2\pi r_w^2{S}_{s}b}\text{(11)}$$ $$t_D=\frac{Kt}{S_s{r}_w^2}\text{(12)}$$ $$h_D=\frac{4\pi Kb}{Q}\left(h_0-h\right)\text{(13)}$$

where

• $b$ is aquifer thickness [L]
• $b^{\prime }$ is block thickness or diameter [L]
• $b_s$ is thickness of fracture skin [L]
• $h$ is head in aquifer [L]
• $h_0$ is static head in aquifer [L]
• $K$ is fracture hydraulic conductivity [L/T]
• $K^{\prime }$ is matrix hydraulic conductivity [L/T]
• $K_i$ is modified Bessel function of second kind, order i
• $K_s$ is fracture skin hydraulic conductivity [L/T]
• $p$ is Laplace transform variable
• $Q$ is pumping rate [L³/T]
• $r$ is radial distance from pumping well to observation well [L]
• $r_c$ is casing radius [L]
• $r_w$ is well radius [L]
• $S_s$ is fracture specific storage [L^-1]
• $S_s^{\prime }$ is matrix specific storage [L^-1]
• $S_f$ is fracture skin factor [dimensionless]
• $S_w$ is wellbore skin factor [dimensionless]
• $t$ is elapsed time since start of pumping [T]

Notes

1. Moench (1988) extended the method to include partial penetration and anisotropy based on the work of Dougherty and Babu (1984). The anisotropy ratio, $K_z/K_r$, refers to the hydraulic conductivity of the fracture system.
2. In the Laplace transform solution, $S_w$ is limited to positive values; however, using the effective well radius concept, we also may simulate a negative skin (Hurst, Clark and Brauer 1969).
3. If you enter a radius for downhole equipment, AQTESOLV uses the effective casing radius instead of the nominal casing radius in the equations for this solution.
4. Refer to the Moench (1997) solution for the equations relating to delayed response in an observation well.

Data Requirements

• pumping and observation well locations
• pumping rate(s)
• observation well measurements (time and displacement)
• casing radius and wellbore radius for pumping well(s)
• downhole equipment radius (optional)
• aquifer thickness
• thickness of slab blocks or diameter of spherical blocks

Solution Options

• large-diameter pumping wells
• partial penetrating wells
• hydraulic conductivity anisotropy
• variable pumping rates
• multiple pumping wells
• multiple observation wells
• boundaries

Estimated Parameters

• $K$ (fracture hydraulic conductivity)
• $S_s$ (fracture specific storage)
• $K^{\prime }$ (matrix hydraulic conductivity)
• $S_s^{\prime }$ (matrix specific storage)
• $S_w$ (wellbore skin factor)
• $S_f$ (fracture skin factor)
• $r\left(w\right)$ (well radius)
• $r\left(c\right)$ (casing radius)

Curve Matching Tips

• Use radial flow plots to help diagnose wellbore storage.
• Use the Cooper and Jacob (1946) solution to late-time data to obtain preliminary estimates of aquifer properties.
• Match early-time data affected by wellbore storage by adjusting $r\left(c\right)$ with parameter tweaking.
• If you estimate $r\left(c\right)$ for the test well, the estimated value replaces the nominal casing radius and AQTESOLV still performs the correction for downhole equipment.
• Choose Match>Visual to perform visual curve matching using the procedure for type curve solutions.
• Use active type curves for more effective visual matching with variable-rate pumping tests.
• Select values of $S_f$ from the Family and Curve drop-down lists on the toolbar.
• Use parameter tweaking to perform visual curve matching and sensitivity analysis.
• Perform visual curve matching prior to automatic estimation to obtain reasonable starting values for the aquifer properties.

Benchmark

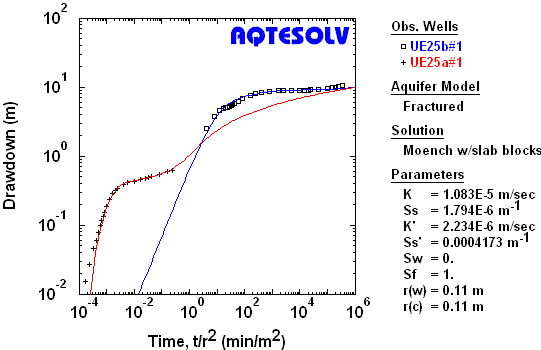
References

Moench, A.F., 1984. Double-porosity models for a fissured groundwater reservoir with fracture skin, Water Resources Research, vol. 20, no. 7, pp. 831-846.

Moench, A.F., 1988. The response of partially penetrating wells to pumpage from double-porosity aquifers, Proceedings of the International Conference on Fluid Flow in Fractured Rocks, Atlanta, GA, May 16-18, 1988.