Hantush Solution for Wedge-Shaped Confined Aquifers
- Assumptions
- Equations
- Data requirements
- Solution options
- Estimated parameters
- Curve matching tips
- References
Related Solution Methods
Additional Topics
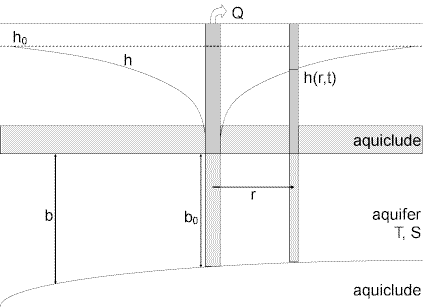
 A mathematical solution by Hantush (1962) may be used to estimate hydraulic properties (transmissivity and storativity) of wedge-shaped nonleaky confined aquifers from constant- or variable-rate pumping tests.
A mathematical solution by Hantush (1962) may be used to estimate hydraulic properties (transmissivity and storativity) of wedge-shaped nonleaky confined aquifers from constant- or variable-rate pumping tests.
Interpretation involves matching type curves for the Hantush solution to drawdown data collected during a pumping test in a wedge-shaped aquifer. Hantush's solution assumes fully penetrating wells and a nonleaky confined aquifer whose thickness varies exponentially.
You are not restricted to constant-rate tests with the Hantush solution. Using the principle of superposition in time, AQTESOLV can simulate variable-rate and recovery tests with this method.
Assumptions
- aquifer has infinite areal extent
- aquifer is homogeneous and isotropic
- control well is fully penetrating
- flow to control well is horizontal
- aquifer is nonleaky confined
- flow is unsteady
- water is released instantaneously from storage with decline of hydraulic head
- diameter of control well is very small so that storage in the well can be neglected
- the thickness of the aquifer varies exponentially in the direction of flow
- the rate of change in aquifer thickness in the direction of flow does not exceed 0.20
- the influence of pumping has not extended beyond the section of the aquifer at which the tangents of the maximum angles of dip of the confining beds are greater than 0.20
Equations
Hantush (1962) derived an equation which predicts drawdown in a wedge-shaped confined aquifer in response to pumping:
where
- is aquifer thickness [L]
- is aquifer thickness at the pumped well [L]
- is pumping rate [L³/T]
- is radial distance from pumping well to observation well [L]
- is drawdown [L]
- is storativity [dimensionless]
- is elapsed time since start of pumping [T]
- is transmissivity [L²/T]
- is coordinate direction [L]
- is x-coordinate of the pumped well [L]
- is a variable of integration
Ordinarily, the slope on the base of the confined aquifer varies exponentially in the x direction and is constant in the y direction. The slope orientation angle measures the orientation of the slope in relation to the x-coordinate axis and is measured counterclockwise from the positive x-coordinate axis to the vector oriented in the updip direction of the slope. The parameter is a constant defining the variation in thickness of the wedge-shaped aquifer.
The solution assumes that the change in aquifer thickness, , as a function of distance meets the following criterion:
and that the duration of pumping does not exceed the following limitation:
Hydrogeologists commonly refer to the integral in the drawdown equation as the Hantush well function for leaky aquifers.
Data Requirements
- pumping and observation well locations
- pumping rate(s)
- observation well measurements (time and displacement)
Solution Options
- variable pumping rates
- multiple observation wells
Estimated Parameters
AQTESOLV provides visual and automatic methods for matching the Hantush method to data from pumping tests and recovery tests. The estimated aquifer properties are as follows:
- (transmissivity)
- (storativity)
- (thickness variation parameter)
Curve Matching Tips
- Match the Cooper and Jacob (1946) solution to late-time data to obtain preliminary estimates of aquifer properties.
- Choose Match>Visual to perform visual curve matching using the procedure for type curve solutions.
- Use active type curves for more effective visual matching with variable-rate pumping tests.
- Select values of from the Family and Curve drop-down lists on the toolbar.
- Use parameter tweaking to perform visual curve matching and sensitivity analysis.
- Perform visual curve matching prior to automatic estimation to obtain reasonable starting values for the aquifer properties.
References
Hantush, M.S., 1962. Flow of ground water in sands of nonuniform thickness; 3. Flow to wells, Jour. Geophys. Res., vol. 67, no. 4, pp. 1527-1534.